Chapter 4

Gamma

Imagine you are riding your bicycle and you are pedalling at constant speed. Assuming that you are riding on fairly flat terrain, you can use your bike speed to tell how far you can travel in 20 minutes. What if you are in a rush and need to arrive at your destination sooner? To cover more ground faster, you pedal harder and your speed increases. The question you want to answer is how much did your time until arrival change based on you pedalling harder? While not a perfect analogy, this is similar to the relationship between delta and the next Greek we will discuss – gamma.

What is Gamma? 

We previously discussed delta and highlighted that this is the most important Greek. As a refresher, an option’s delta tells you how much an option’s price will change for every unit of change in the underlying index or stock. For example, assume the delta is 0.32 for an option contract on the Bank Nifty. If the Bank Nifty goes up by 1, then the price of this option will go up by approximately 0.32.

What we also learned was that delta varies by moneyness. In the illustration 1 below, we plot the delta values for a hypothetical option with a strike price of 100. As you move from an underlying price of 95 to 100, the delta goes from around 0.05 to 0.50. You can interpret this as the option becoming more sensitive to the change in underlying price. When the underlying is priced at 95, the option will increase in value by ~0.05,  if the underlying price goes to 96. However, if the underlying is priced at 100, then the option will increase in value by ~0.50 if the underlying price goes to 101.

Illustration 1

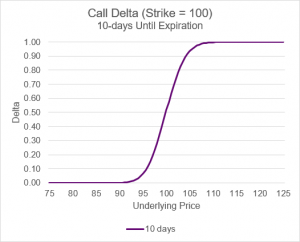
As a trader, you may want to understand how this sensitivity to the underlying’s price will change as the underlying goes up in price. Gamma is the solution. Gamma is the rate of change of delta for a one-unit change in the underlying.

Illustration 2 is a screenshot of the Upstox Option Chain. The third column from the left has the values for gamma. This particular option chain is the Nifty 50 for call options expiring within one week. As you can see, the values of gamma are small; especially when you compare them to delta, theta, and vega. 

While the values are small, you can still see that they increase from 0.001 to 0.002 as the strike price goes from out-of-the-money to at-the-money. As the options become more in-the-money, gamma goes down from 0.002 to 0.001. 

Keep in mind, we are rounding to the third decimal place and the changes will occur more granularly. 

Illustration 2

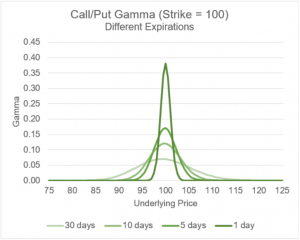
Illustration 3 is a plot of gamma values at different levels of moneyness and across different expirations. There are three key callouts from this chart:

1. An option will be more sensitive to price changes in the underlying if the underlying is close to being at-the-money.
   • If an option is deep in-the-money or deep-out-of-the-money, delta won’t change much as an underlying’s price changes.
   • However, if an option is close to being at-the-money, gamma will be high leading to large changes in delta as the underlying moves in price.
2. As time until expiration increases, an option’s gamma becomes more sensitive to the underlying’s price change if the option is close to being at-the-money.
   • If an option is out-of-the-money or in-the-money, then gamma will have the most impact if there is more time until expiration.
3. The gamma values for calls and puts are the same.

Illustration 3

To conclude: 

• Gamma is the rate of change of delta for a one-unit change in the underlying.
• Use this to see by how much the option price will change with for every unit increase in the price of the underlying.
• Values of gamma are smaller than delta, theta, and vega.
• Gamma increases as strike price moves from out-of-the-money to at-the-money.
• Gamma decreases as options become more in-the-money.
• Gamma values for calls and puts are the same.

From a practical perspective, gamma is usually most watched by professional traders or market makers as they look to hedge option positions with the underlying equities by using delta. Since these market makers are critically focused on delta, they need to monitor gamma closely since it gives them insights into changes in delta. 

On a day-to-day basis, gamma shouldn’t drastically impact your trading. However, if you are trading an option on expiration day (or the day before expiryprior), gamma can significantly impact the your P&L of your trades. This is especially the case if the option you own is at-the-money and if the underlying is having a particularly volatile day.

That brings us to the end of the course on Options and Options Strategies. Do take one last quiz to test yourself. We round off with the Epilogue which will give you a Trader’s perspective on Options.