Long Call
Introduction to Long Calls
To understand this strategy, let us go back to the analogy we used in the chapter on Call options.
We had assumed the following - You bought a flat costing ₹1 crore from the owner. To secure your interest in the property, you paid 5% of the cost as a ‘token.’ So, for a sum of ₹5 lakh, you purchased rights to a property worth ₹1 crore. This allowed you to buy it at a future date at the predetermined price of ₹1 crore.
In our example, the government had started work on a new metro line six months after you purchased the property, which upped its asking rate.
Now let’s assume instead that you knew about this beforehand and had bought the property expecting prices to go up. You plan to sell the property at a profit and exit your investment. That is exactly what you would be doing if you were to go long on a call option in the stock market.
Why would a trader use a long call?
You buy or ‘go long’ on a Call Option if you believe two things:
- The underlying stock or index will move upward AND
- You expect this upward move to occur prior to the selected expiration date of the option.
The benefit of purchasing a call option instead of the underlying is that the cost to enter the position can be substantially lower. For example, if you were bullish on TCS that is currently trading at ₹3,877, the cost to buy 175 shares of this company would be ₹6,78,475.
However, an at-the-money option with 1 month until expiration could be trading at ₹137.00 for a total cost of 23,975 to purchase 175 options.
The drawback is that you are purchasing a contract rather than the actual asset. If you purchased the underlying, you would own that asset until you sell it. By purchasing the call option instead of the underlying, you could end up with something worthless at expiration if the underlying doesn’t move favorably.
How do you construct a long call?
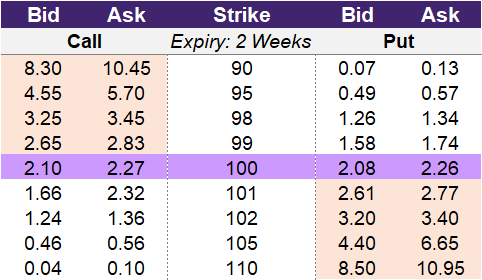
The table below is an example of an option chain with bid and offer prices for 9 strikes associated with calls and puts on one expiration date. In this example data, the underlying is trading at 100 so the at-the-money strike price is 100.
If you were ready to place a trade for a long call option, you would look to the left side of the option chain for the call option section. Since you would be buying, you will likely pay closer to the ask price rather than the bid price. The ask price here is the ‘market price to buy’. You could select from any of the strikes listed. For example, if you wanted to buy the call option with the strike price of 100, this would cost you 2.27, which is the asking price for this option contract.
Illustration 1
 Source: Upstox
Source: UpstoxWhat is the max profit of a long call?
Key Formula:
- Long Call Max Profit = Unlimited – Premium Paid
The maximum gain you can have with a long call option is theoretically unlimited. This is because the price of a stock or index can theoretically go to infinity. However, this is an unlikely scenario, and as a trader, you should more accurately assess your gain-to-loss ratio using historical data that matches the time period of the option.
For example, if you purchase an option with 14 days to expiration and plan to give the option up to the full 14 days to become profitable, you could refer to historical 14-day return distributions to understand what is a more realistic maximum gain. In the table below, we show the historical 14-day returns for the Nifty. You could define your “realistic max gain” as a 1 out of 20 scenario or top 5% of historical returns. This means that 5.2% could be a more reasonable estimate of max gain instead of unlimited potential gain. With a number like 5.2%, you can then more easily assess return versus risk.
Illustration 2: Nifty50 14-Day Historical (10-yr) Returns
| Top % | Return | Probability |
|---|---|---|
| Top 1% | 8.5% | 1 out of 100 |
| Top 2% | 6.7% | 1 out of 50 |
| Top 5% | 5.2% | 1 out of 20 |
| Top 10% | 4.0% | 1 out of 10 |
Similarly, if you buy an option with 14 days until expiration but only plan to trade intraday, you could look at daily open-to-close returns or close-to-close return distributions to assess the realistic maximum gain.
How much can you lose trading a long call?
Key Formula:
- Long Call Max Loss = Premium Paid
The maximum loss is capped at the price you purchased the option for. In the option chain example above, if you selected the at-the-money strike of 100, the premium or cost that you paid to buy the call option is 2.27. This cost is the maximum you can lose in this trade.
What is the breakeven point when entering a long call?
Key Formula:
- Long Call Break-even Point = Strike Price + Call Option Cost
The break-even point is the strike price plus the cost to buy the call option. Using the example data from Illustration 1, if you bought the 100-strike, it would cost you 2.27 (the ask price). The break-even price would be 102.27 (100 + 2.27). In our example, the underlying is trading at 100.
For the long call to break even, the underlying would need to rise from 100 to 102.27. This would be an increase of 2.27% over the current price of 100. By comparison, if you purchased the 102-strike, it would cost you 1.36, which is less expensive than the cost of the 100-strike. However, the breakeven point of the 102-strike call option is 103.36 (102 + 1.36). While the 102-strike is 40% cheaper than the 100-strike, the underlying needs to move up 3.36% instead of only needing to rise by 2.27%.
What is the profit formula for a long call?
Key Formula:
- Long Call Payoff = Max(0, Underlying Price – Strike Price)
- Long Call Profit = Max(0, Underlying Price – Strike Price) – Option’s Cost
The formula for the payoff of a long call is the maximum of 0 or the difference between the underlying price and the strike price. The profit formula for a long call is the long call’s payoff minus the cost to purchase the option.
What is the payoff diagram for a long call?
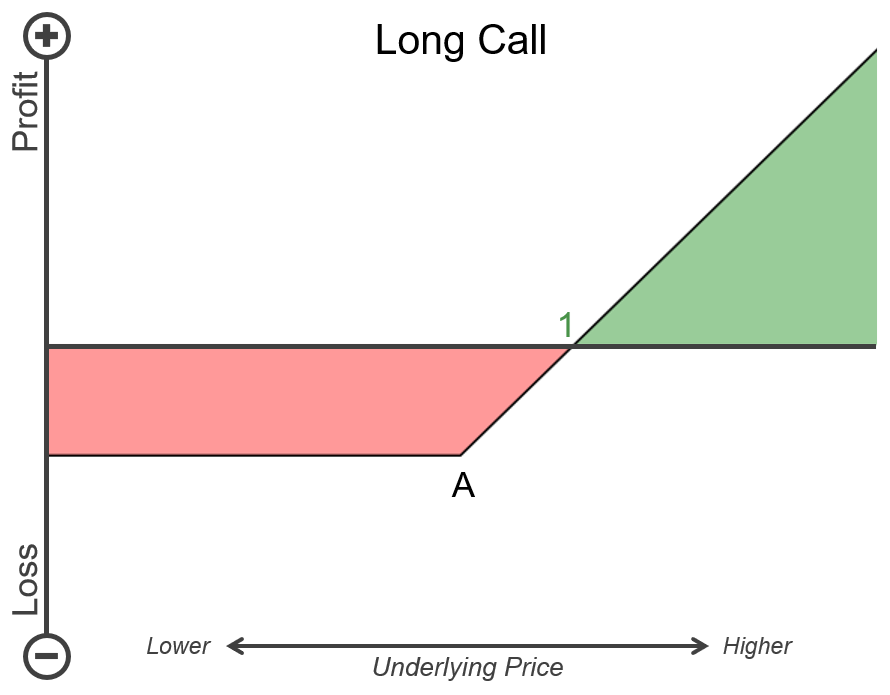
Below is an example P&L diagram for a long call. Point A on the chart is the strike price selected for the long call. Point 1 is the breakeven point for this particular strategy. The vertical axis is the profit and loss where the higher up represents more profit and further down is a higher loss. The horizontal is the underlying price. Further to the right is a higher underlying price and further to the left is a lower underlying price. Areas that are shaded green are when the long call will have a positive payoff and areas shaded red are when the long call will have a negative payoff.
If the price of the underlying stays at or above (to the right) of the breakeven point at point 1, then the trader will have a profitable trade. If the price of the underlying is between the strike price (A) and the breakeven point (1) on expiry, then the trade will result in a loss but not the max loss. The max loss will occur if the underlying price expires below (to the left) of the strike price (A).
Illustration 3
 Source: Upstox
Source: UpstoxWhat is the point of max profit for a long call?
Key Formula:
- Price of Underlying = ∞
The long call option doesn’t have a specific point of maximum profitability because the max profit isn’t capped. If the underlying keeps going up, the trader will earn more due to the rising value of the call option. Technically, the max profit of a long call occurs if the underlying rises to infinity.
What is the point of max loss for a long call?
Key Formula:
- Price of Underlying ≤ Strike Price
The point of maximum loss for a long call occurs if the underlying doesn’t move at all or falls. If this happens, the long call would have no intrinsic value on expiration resulting in the trading not having a payoff from the long call option. The trader will still have paid the premium to enter into the strategy which is the max loss for a long call.
Summary
- Go long on a call option if you believe the price of the underlying will increase before expiry.
- The option becomes worthless if the underlying price doesn't increase.
- Calls are listed to the left of the option chain.
- You pay the 'ask price' to buy an option.
- Maximum gain on a long call option is theoretically unlimited.
- Maximum loss is capped at the ask price.
Is this chapter helpful?
- Home/
- Long Call